Arnaud Bodin

Articles

See also on arXiv or MathSciNet.

Preprints

Publications

Around the gcd of the values of two polynomials

with Christian Drouin, to appear in American Math. Monthly

We propose a mathematical walk around the gcd of the values A(n) and B(n) of two polynomials evaluated at an integer n. This is an opportunity to use a very powerful tool: the resultant.

Combinatorial study of morsifications of real univariate singularities

with Evelia Rosa García Barroso, Patrick Popescu-Pampu and Miruna-Stefana Sorea, Math. Nachrichten, 2024

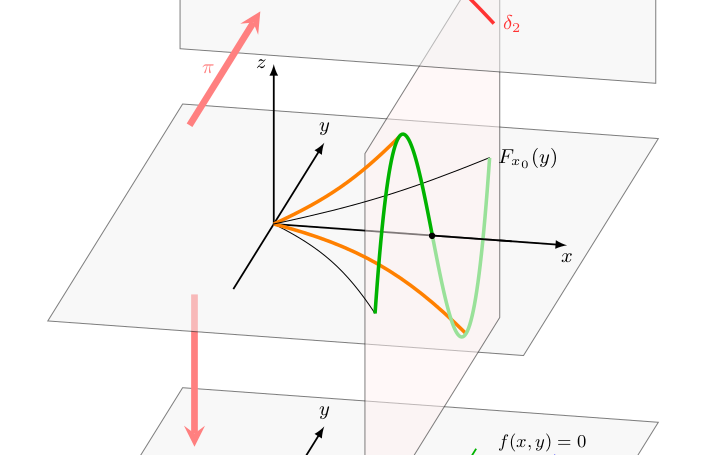
We study a broad class of morsifications of germs of univariate real analytic functions. We characterize the combinatorial types of the resulting Morse functions, via planar contact trees constructed from Newton-Puiseux roots of the polar curves of the morsifications.

Poincaré-Reeb graphs of real algebraic domains

with Patrick Popescu-Pampu and Miruna-Stefana Sorea, Revista Matemática Complutense, 2023

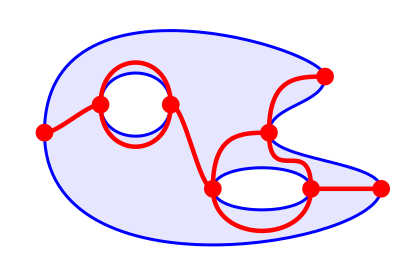
An algebraic domain is a closed topological subsurface of a real affine plane whose boundary consists of disjoint smooth connected components of real algebraic plane curves. We study the non-convexity of an algebraic domain by collapsing all vertical segments contained in it: this yields a Poincaré-Reeb graph, which is naturally transversal to the foliation by vertical lines. We show that any transversal graph whose vertices have only valencies 1 and 3 and are situated on distinct vertical lines can be realized as a Poincaré-Reeb graph.

Coprime values of polynomials in several variables

with P. Dèbes, Israel J. Math., 257, 2023

Given two polynomials P(x), Q(x) in one or more variables and with integer coefficients, how does the property that they are coprime relate to their values P(n), Q(n) at integer points n being coprime? We show that the set of all gcd (P(n), Q(n)) is stable under gcd and under lcm. A notable consequence is a result of Schinzel: if in addition P and Q have no fixed prime divisor (i.e., no prime dividing all values P(n), Q(n)), then P and Q assume coprime values at “many” integer points. Conversely we show that if “sufficiently many” integer points yield values that are coprime (or of small gcd) then the original polynomials must be coprime. Another noteworthy consequence of this paper is a version over the ring of integers of Hilbert’s irreducibility theorem.

The Hilbert-Schinzel specialization property

with P. Dèbes, J. König and S. Najib, Crelle’s Journal, 785, 2022

We establish a version “over the ring” of the celebrated Hilbert Irreducibility Theorem. Given finitely many polynomials in k+n variables, with coefficients in Z, of positive degree in the last n variables, we show that if they are irreducible over Z and satisfy a necessary “Schinzel condition”, then the first k variables can be specialized in a Zariski-dense subset of Z^k in such a way that irreducibility over Z is preserved for the polynomials in the remaining n variables. The Schinzel condition, which comes from the Schinzel Hypothesis, is that, when specializing the first k variables in Z^k, the product of the polynomials should not always be divisible by some common prime number. Our result also improves on a “coprime” version of the Schinzel Hypothesis: under some Schinzel condition, coprime polynomials assume coprime values. We prove our results over many other rings than Z, e.g. UFDs and Dedekind domains for the last one.

Bilipschitz equivalence of polynomials

Mathematische Nachrichten, 294, 2021

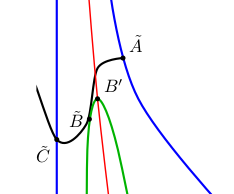
We study a family of polynomials in two variables having moduli up to bilipschitz equivalence: two distinct polynomials of this family are not bilipschitz equivalent. However any level curve of the first polynomial is bilipschitz equivalent to a level curve of the second.

The Schinzel hypothesis for polynomials

with P. Dèbes and S. Najib, Transaction AMS, 373, 2020

The Schinzel hypothesis is a famous conjectural statement about primes in value sets of polynomials, which generalizes the Dirichlet theorem about primes in an arithmetic progression. We consider the situation that the ring of integers is replaced by a polynomial ring and prove the Schinzel hypothesis for a wide class of them: polynomials in at least one variable over the integers, polynomials in several variables over an arbitrary field, etc. We achieve this goal by developing a version over rings of the Hilbert specialization property. A polynomial Goldbach conjecture is deduced, along with a result on spectra of rational functions.

Prime and coprime values of polynomials

with P. Dèbes and S. Najib, L’enseignement mathématique, 66, 2020

The Schinzel Hypothesis is a celebrated conjecture in number theory linking polynomials and prime numbers. In the same vein, we investigate the common divisors of values of polynomials and establish a “coprime Schinzel Hypothesis”. We deduce a version “modulo an integer” of the original Hypothesis, and as special cases, the Goldbach conjecture and the Twin Primes conjecture, again modulo an integer.

with M. Borodzik, Israel J. Math., 227, 2018

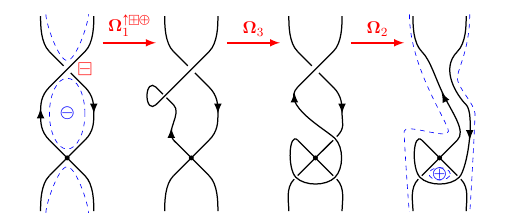
For a smooth complex curve we consider the link Lr obtained by intersecting the curve with a sphere of radius r. We prove that the diagram Dr obtained from Lr by a complex stereographic projection satisfies topological equalties. As a consequence we show that if Dr has no negative Seifert circles and Lr is strongly quasipositive and fibered, then the Yamada–Vogel algorithm applied to Dr yields a quasipositive braid.

Families of polynomials and their specializations

with P. Dèbes and S. Najib, J. Number Theory, 170, 2017

For a polynomial in several variables depending on some parameters, we discuss some results to the effect that for almost all values of the parameters the polynomial is irreducible. In particular we recast in this perspective some results of Grothendieck and of Gao.

The braid group of a necklace

with P. Bellingeri, Math. Z., 283, 2016

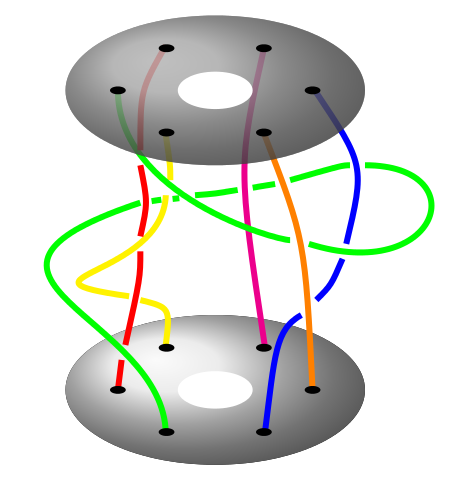
We study several geometric and algebraic properties of a necklace: a link composed with a core circle and a series of circles linked to this core. We first prove that the fundamental group of the con- figuration space of necklaces is isomorphic to the braid group over an annulus. We then defne an action on the fundamental group of the configuration space of the necklaces to the automorphisms of the free group and characterize these automorphisms among all automorphisms of the free group.

Topology of generic line arrangements

Asian J. of Math., 19, 2015

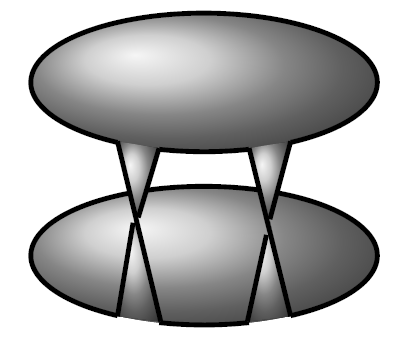
Our aim is to generalize the result that two generic complex line arrangements are equivalent. In fact for a line arrangement A we associate its defining polynomial f= prod_i (a_ix+b_iy+c_i), so that A= (f=0). We prove that the defining polynomials of two generic line arrangements are, up to a small deformation, topologically equivalent. In higher dimension the related result is that within a family of equivalent hyperplane arrangements the defining polynomials are topologically equivalent.

Waring problem for polynomials in two variables<

with Mireille Car, Proc. American Mathematical Society, 141, 2013

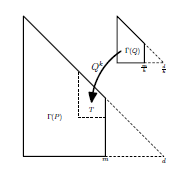
We prove that all polynomials in several variables can be decomposed as the sum of k-th powers: P(x_1,…,x_n) = Q_1(x_1,…,x_n)^k+ … + Q_s(x_1,…,x_n)^k, provided that elements of the base field are themselves sum of k-th powers. We also give bounds for the number of terms s and the degree of the Q_i^k. We then improve these bounds in the case of two variables polynomials to get a decomposition P(x,y) = Q_1(x,y)^k+ … + Q_s(x,y)^k with deg Q_i^k less than deg P + k^3 and s that depends on k and ln(deg P).

Specializations of indecomposable polynomials

with Guillaume Chèze and Pierre Dèbes, Manuscripta Mathematica, 139, 2012

We address some questions concerning indecomposable polynomials and their behaviour under specialization. For instance we give a bound on a prime p for the reduction modulo p of an indecomposable polynomial P(x) in Z[x] to remain indecomposable. We also obtain a Hilbert like result for indecomposability: if f(t_1,…,t_r,x) is an indecomposable polynomial in several variables with coefficients in a field of characteristic p=0 or p>deg(f), then the one variable specialized polynomial f(t_1+a_1 x,…,t_r+a_r x,x) is indecomposable for all (t_1,…, t_r, a_1,…,a_r) i the closure of k^{2r} off a proper Zariski closed subset.

Integral points on generic fibers

J. London Mathematical Society, 81, 2010

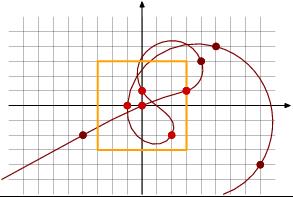
Let P(x,y) be a rational polynomial and k in Q be a generic value. If the curve (P(x,y)=k) is irreducible and admits an infinite number of points whose coordinates are integers then there exist algebraic automorphisms that send P(x,y) to the polynomial x or to x^2-dy^2, d in N. Moreover for such curves (and others) we give a sharp bound for the number

Decomposition of polynomials and approximate roots

Proc. American Mathematical Society, 138, 2010

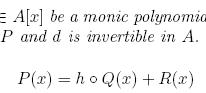
We state a kind of Euclidian division theorem: given a polynomial P(x) and a divisor d of the degree of P, there exist polynomials h(x),Q(x),R(x) such that P(x) = h(Q(x)) +R(x), with deg h=d. Under some conditions h,Q,R are unique, and Q is the approximate d-root of P. Moreover we give an algorithm to compute such a decomposition. We apply these results to decide whether a polynomial in one or several variables is decomposable or not.
See the library at the end of this page for examples of computations.

Generating series for irreducible polynomials

Finite Fields and Applications, 16, 2010

We count the number of irreducible polynomials in several variables of a given degree over a finite field. The results are expressed in terms of a generating series, an exact formula and an asymptotic approximation. We also consider the case of the multi-degree and the case of indecomposable polynomials. See the examples of computations below.

Others

Quelques contributions à la topologie et à l’arithmétique des polynômes

Habilitation Thesis, University Lille 1, July 2008

Les polynômes de plusieurs variables sont présents sous de nombreuses formes en géométrie. L’exemple qui vient immédiatemment à l’esprit est celui d’une courbe algébrique, définie comme le lieu des points qui annulent un polynôme…

Fibres et entrelacs irréguliers à l’infini

Ph.D. Thesis, University Toulouse 3, December 2000

Computations

Last modification : September 2024